IBPA Correspondence, November 2013: The Great Victory Point Debate by John Carruthers
It’s fair to say that I’ve received more correspondence (both in terms of frequency and volume!) on this issue than on any other since I began as editor. If we published it all, it would consume more than a normal month’s Bulletin – I’ve added four pages this month to deal with it. The next few pages will try to present both sides fairly.Even so, I’ve had to abridge the correspondence to make it fit our space. The unabridged versions of both Ron Klinger’s and Ernesto d’Orsi’s texts can be found on the IBPA website and the links have been emailed to members.
First, Ron Klinger asks some penetrating questions about the scale. The responses come from a bridge theorist in Sydney (hereafter SBT), Michael Wilkinson, and the WBF Scoring Committee (hereafter WSC). The WBF Scoring Committee consists of: Henry Bethe, Bart Bramley, Peter Buchen, Mauricio Di Sacco, Manolo Eminenti, Max Bavin (co-chairman), Ernesto d’Orsi (chairman). Their response was prepared by Peter Buchen.
1. Why have we decided to add to the complexity of scoring by introducing a scale with two decimal places? How does the average bridge player make any sense of this approach? Almost all would consider it incomprehensible? Would we report a cricket match as one side being 7.43 wickets for 286.79 runs? Or a football match as 3.72 goals to 1.68? Or a rugby match as 33.55 to 12.31? Or a tennis match as 3.52 sets to 1.44?
SBT: It’s difficult to come up with any vaguely sensible rebuttal to this.
WSC: It is somewhat disingenuous to select cricket, football, rugby and tennis to argue the inappropriateness of decimal scoring in sports. Such cherrypicking ignores the whole gamut of sports, many of them Olympic, which already use decimal scoring. Snow-boarding and skydiving contests employ scoring to one decimal place; running, swimming and high diving to two decimal places; gymnastics to three decimal places; motor racing to four decimal places.
IBPA Ed.: Some of the examples from WSC are times or distances expressed as decimals and are thus easy to understand.
2. Why is the first IMP in a match worth twice as much as the 22nd? Why is it more meritorious to win 10 matches by 5 IMPs (118.5 VPs) than five matches by 10 IMPs and 5 draws (117.15 VPs)?
SBT: To my mind there is something more meritorious about winning all your matches by a small margin than drawing some matches (or indeed losing them by a small margin) and thrashing some of the weaker teams – so the current WBF scale does to a small extent reduce the importance of bunny bashing. See below though – my suggested scale does a far better job of achieving this goal.
WSC: The Scoring Panel has taken the reasonable view that winning a match, by whatever margin, should be rewarded with a higher VP score. This was not the case for the old WBF scales where a draw could be awarded even if there was a 3 or 4 IMP difference.
3. A slam on a finesse is a 50-50 bet. This is recognised in basic duplicate scoring (480 vs 980, 450 and -50, 500 in each case and 11 IMPs in each case; 680 vs 1430 or 650 and -100, 750 in each case and 13 IMPs in each case). Under the new VP scale the strategy for a team trailing, by 20, 30, 40 or 50 IMPs is to bid all slams on a finesse, since the reward for making exceeds the loss for losing. Similarly, the strategy for a team ahead by the same margins is not to bid a slam on a finesse; the potential loss is greater than the potential gain. The new scoring scale thus impacts on the mathematics of the game. To a lesser extent, the same applies to bidding or not bidding games on a finesse.
SBT: It’s difficult to come up with any vaguely sensible rebuttal to your underlying point. The scale I suggest does have an element of this problem – but it’s less clearcut than with the WBF scale. Incidentally, this is my main objection to Butler scoring – it distorts the basic odds for bidding decisions. Interestingly, you are wrong though on your statement about the strategy for a team in the lead – knowing that their opponents will bid all slams on a finesse they should in fact also bid the slam on a finesse to flatten the board and retain their lead – and then we’re playing poker.
WSC: There is nothing new in a bidding strategy that adopts an odds-against action when down a significant margin in a match. The new WBF scale does indeed impact on the odds for a given tactical action dependent on the current IMP17 margin. For the case of the 11 IMP slam swing above, when both teams employ an optimal game-theoretic strategy (ie. they both bid the slam half the time) the expected VP gain for the team behind is positive, while for the team ahead it is negative. The maximum difference is less than 0.25 VPs on the new scale, so such considerations should have little impact. Incidentally, even on the old scale there would be similar tactics in play, with variable differences in VPs.
4. Why is the cut-off for a 16-board match deemed to be worth 3.75 IMPs per board, but for a 10-board match, it is 4.8 IMPs per board?
SBT: This one actually makes a lot of sense – there have been studies done which show that the variance at IMPs is proportional to the squareroot of the number of boards being played – so for a longer match the cutoff should indeed be lower in IMPs/board terms.
WSC: Consider a long 96-board match (as is played in the Bermuda Bowl final and other major events). A team that wins such a match by 144 IMPs say would rightly be regarded as having trounced its opponents. Now consider a four board play-off where one team wins by 6 IMPs. No-one would consider this to be a big win, because it might have been gained in a single lucky deal. Yet both results average 1.5 IMPs per board. Clearly a blitz win should not be based on a constant number of IMPs per board, but rather: the more boards in play, the lower the number of IMPs per board for a blitz. The Scoring Panel defines the blitz win as roughly two standard deviations above the expected mean for two equally matched teams. About 96% of all results will fall within two standard deviations. Based on a large data set, the standard deviation was found to be about 7.5 IMPs per board. Statistical theory then predicts that two standard deviations for N boards will be 15pN IMPs and the average IMPs per board for a blitz will then be 15=pN. This formula yields the following results:
| No. Boards = N | Blitz IMPs/Board | |
| 4 | 7.5 | |
| 10 | 4.74 | |
| 16 | 3.75 | |
| 96 | 1.53 |
5. Why are we adopting a scale incomprehensible to the public, when we can achieve the same (or perhaps better) by means of a straightforward and understandable scale, one where every IMP counts and every IMP is equal? Attached are three files which cover the qualifying rounds of the 2013 Bermuda Bowl, Venice Cup and D’Orsi Trophy. (These are not reprinted here, but are in Ron’s document on the website. – Ed.) The left hand column contains the finishing order of the teams using the new WBF scale. The right-hand column has the total VPs for each team based on a VP scale of 100, where each side starts with 50 VPs. A tie is 50-50. Winners add their net IMPs to 50, cut-off at 100. Losers deduct their IMPs from 50, minimum 0. You will see that the top eight places are exactly the same under both scales (with a slight shuffle) and the total finishing order of the 22 team is almost the same. When reporting the results under such a scale, it would be easy for the public to understand a 73-27 or 91-9 or 54-46 win. Given that the outcome of both scales is effectively the same in selecting the quarter-finalists, what benefits do the new VP scale provide? (Incidentally, it would be prudent to check my maths!)
SBT: There is a good reason for not capping the score for the losers – the reason you outlined above about it changing the bridge maths – once you are trailing by 50 IMPs there is no downside at all in taking a “swingy” action – you are getting anyway. I for one would heartily approve of your scale so long as the negative cap was removed. Although you would perhaps dislike the added complexity – I think a slightly better approach is;
1 IMP = 1 VP for the first n IMPs (where n is dependent on the length of the match)
1 IMP = 0.5 VPs for the next n imps
1 IMP = 0.1 VPs for the next n IMPs for the winners, but with no cap on the losers’ score.
In the case of a 16 board match n = 25 feels about right to me.
WSC: There are two reasons that the suggested 100 VP scale above should be rejected. First, it makes no allowance for the number of boards in play and as we saw in our reply to Q4. above, this is an important element in any IMP to VP conversion. How many scores of 100–0 would you see in a 10 board match requiring an average of 10 IMPs per board? Correcting this anomaly would mean having the highly undesirable feature of a different blitz VP for each match with a different board quota. Secondly the proposed linear scale implies each IMP up to the maximum is worth the same increment in VP’s. We have explained in our response to Q2. why this is also undesirable.
IBPA Ed.: No one is suggesting that the 0-100 scale be used for all matches regardless of length. For example, a 10-board match might use a 0-60 VP scale with 30 IMPs a blitz.
6. Someone might care to conduct the same exercise for previous World Championships by scoring the qualifying rounds under both the new WBF scale and the suggested 100 VP scale.
SBT: At some point I might rescore some events but not at 12.30 a.m.18
WSC: The WBF Scoring Panel are currently conducting statistical analyses of past World Championships with a view to perhaps fine tuning some of the parameters of the new WBF scale. For the reasons stated above, it is unlikely that the WBF would adopt a scale on the basis suggested by Ron Klinger.
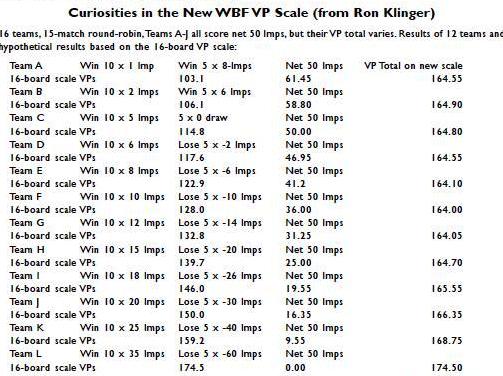
Although all teams scored the same number of net Imps, the semi-finalists would again be Teams L, K, J, I. It’s not
the number of Imps you score, but how you score them that counts.
Team X Win 10 x 24 Imps Lose 5 x -40 Imps Net 40 Imps
16-board scale VPs 157.4 9.50 166.90
Team Y Win 10 x 23 Imps Lose 5 x -39 Imps Net 40 Imps
16-board scale VPs 155.6 10.15 165.70
Thus Team X, with net 40 Imps, again finishes third, ahead of many teams that scored 50 Imps, but in different fashion. Team Y, with net 35 Imps, comes fourth ahead of many with net 50 Imps.
Dilip Gidwani wades in much more succinctly:
Dear John,
The conversion of IMPs to VPs is a formula that has been in practice over years. The scales of 25-5 and 20-0 are an outcome of this factoring process. Factoring IMPs to a VP scale seemingly favours teams who lose by huge margins but still have a chance to recoup from one bad session. Once applied uniformly, factoring is a fair measure of reward for victory and penalty for loss. Nevertheless … the ease of the 25-5 scale for journalists is without any doubt, but the 0.00-20.00 scale, although difficult for journalists (and players alike) to remember (as it involves four digits) is however, a fairer scale in terms of reward/penalty for win/loss. This aspect has been researched extensively by statisticians the world over. However, it would be interesting to see how our executive debates this matter.
Dilip Gidwani, Mumbai
The IBPA Executive discussed the matter at its meeting in Bali and in the AGM. Our conclusion was that the new scale,
however fair and accurate, is not good for bridge and especially not good for bridge reporting. These views have been made known to the WBF. – Ed.
Esta entrada también está disponible en: Spanish

